2. Internal Expansion

More needs to be said about the choriambic dimeter. This octosyllable is the most primitive form of regular dimeter inherited by Greek. In its pattern we see the preservation of a clear metrical delineation between opening and closing, with an unfixed rhythm in the former and a fixed rhythm in the latter. The similarity here with the 4 + 4 delineation of opening + closing in the Indic octosyllable [1] is indeed striking. Furthermore, the opening + closing delineation of the choriambic dimeter is reinforced by an important constraint, which becomes apparent when we examine all the possible permutations in the opening: (Triple and quadruple shorts are not likely to have been inherited by Greek [2] and thus have not been listed.) The only rhythmical pattern excluded from the opening is – ⏑ ⏑ –, that is, the choriamb of the closing. [3] It appears that the constraint here is freedom: the opening of the choriambic dimeter must be free, and therefore {37|38} it is not allowed to be a choriamb itself.

Corresponding to the choriambic dimeter, there are residual attestations of what looks like an iambic dimeter with free rhythm in the opening: Watkins has surveyed this type and designated it as the ‘irregular Glyconic’. [4] If syllable 4 had been generalized as short and consequently syllable 3 had been generalized as long (to avoid a triple short), the result would be the Glyconic so that the designation ‘irregular Glyconic’ is well founded. Watkins must have chosen this term instead of ‘iambic dimeter’ because the latter is traditionally applied to the regularized unit where the opening pattern mirrors the closing pattern. Note, however, that this type of octosyllable preserves an archaism lost even by the primitive residual ‘irregular Glyconic’: in syllable 5 (and consequently in syllable 1), there has been no obligatory generalization of short as against long.

In the iambic dimeter, the opening/closing {38|39} symmetry results in the synchronic perception of a metrical segment ⏓ – ⏑ –, the iamb. In the choriambic dimeter, by contrast, the opening/closing asymmetry triggers the segmentation – ⏑ ⏑ –, the choriamb. Both iamb (ia) and choriamb (ch) figure in the constitution of Greek trimeter.

As I will argue in detail, [5] trimeter results from the conflation of dimeter components: In the case of iambic trimeter, where opening equals closing formally, this complex structure is collapsed into a simplex structure: The middle component is overtly neither an opening nor a closing: it is simply an iamb, which may be perceived simply as an insertion between two other iambs. Here we see the start of internal expansion.

The principle of inserting a single ia between ia ia is a mechanism so simple that it could have easily inspired a stylistic elaboration: insert a double ia instead of a single ia. What results is the iambic tetrameter: Within the framework of such a structure, however, {39|40} we find that the iamb (ia) is interchangeable with the choriamb (ch). Consider the four surviving stanzas of Anakreon 388P, where each stanza consists of two tetrameters followed by a dimeter: [6] The same sort of iambic/choriambic interchangeability, which was actually noticed by the metrician Hephaistion, [7] is sporadically attested even in the iambic trimeter. For example, consider the verse-initial formula εἶεν ἀκούω (– ⏑ ⏑ – – …), as used by Aischylos (Libation Bearers 657) and Aristophanes (Peace 663). In the textual tradition of the Iambographoi, we find sporadic instances of – ⏑ ⏑ – even at syllables 5 6 7 8: For example, consider line 4 of Semonides 1D: (For attempts at emendation, see 1.4W and the apparatus.)

Since internal expansion is possible by way of choriambs as well as iambs, the question arises why we fail to find attestations of choriambic trimeter and tetrameter besides iambic trimeter and tetrameter. In other words, why is there no attestation of a genuine closing – ⏑ ⏑ ⏓ preceded regularly by other choriambs? [8] For an answer we must look to the inherent asymmetry of the choriambic dimeter, where the closing – ⏑ ⏑ ⏓ precludes the opening – ⏑ ⏑ –. Such a dimeter with asymmetrical components cannot generate a trimeter with symmetrical components. Where we do see a series of two or more choriambs, the closing will be by necessity an iamb, as in Anakreon 388P above, or a catalectic iamb (ia^ = ⏑ – ⏓), [9] as in the following: A choriambic series or a single choriambic opening may have a choriambic closing only if the latter is catalectic, as in the following: In such patterns, the inherent asymmetry of choriambic verse has been preserved by way of catalexis. The intact choriambs (– ⏑ ⏑ –) of these verses are not mirrored by the truncated choriamb (ch^ = – ⏑ ⏓) in the closing. [10]

In the so-called polyschematist versification of the poetess Korinna (notably the huge fragment 654P), choriambic dimeters freely alternate with {42|43} Glyconics. Since the same phenomenon is attested in Sappho (96LP), [11] it seems reasonable to agree with Snell [12] and Watkins [13] in their conclusion that these two dimeters were once actually variants of one another. Given the functional and formal affinity between these two types of octosyllable, we are led to ask how the Glyconic dimeter might have undergone internal expansion in terms of the choriambic dimeter. We have already seen that the choriamb may serve as the element of internal expansion in the formation of trimeters and tetrameters from dimeters, as long as there is no overt choriamb in the closing. Since the Glyconic ends not in a choriambic but in an iambic rhythm (… ⏑ – ⏑ ⏓), we have reason to expect internal expansion via choriambs. Exactly where the expansion should occur remains a problem, however.

What makes the Glyconic dimeter the Aeolic meter par excellence is the so-called Aeolic base ⏓ ⏓ that precedes the sequence – ⏑ ⏑ – ⏑ ⏓. As with the initial ⏓ ⏓ ⏓ ⏓ of the choriambic dimeter ⏓ ⏓ ⏓ ⏓ – ⏑ ⏑ ⏓, the initial ⏓ ⏓ of the Glyconic ⏓ ⏓ – ⏑ ⏑ – ⏑ ⏓ must be free and cannot be fixed in any predictable pattern from verse to verse. In transition from Glyconic to Glyconic to Glyconic, the static element – ⏑ ⏑ – ⏑ ⏓ must be preceded by a dynamic {43|44} element ⏓ ⏓ which keeps shifting from – – to – ⏑ to ⏑ – to ⏑ ⏑, in unpredictable order and frequency. In other words, the function of the Aeolic base ⏓ ⏓ was to provide the ingredient of rhythmical variety, but on a level statistically more sedate than the almost distractingly varied permutations of the ⏓ ⏓ ⏓ ⏓ in choriambic dimeter. As the length of free rhythm (⏓ ⏓ ⏓ ⏓) in the choriambic dimeter held its metrical segmentation at 4 + 4, the shortened length of free rhythm (⏓ ⏓) in the Glyconic produced a metrical segmentation at 2 + 6. It is at this juncture that internal expansion in fact occurs: The well-known Asclepiad is a dodecasyllabic trimeter that was generated from an octosyllabic dimeter simply by the insertion of a choriamb (– ⏑ ⏑ –) between the rhythmically free (⏓ ⏓) and fixed (– ⏑ ⏑ – ⏑ ⏓) segments of the Glyconic. In size, the Asclepiad corresponds to the iambic trimeter just as the Greater Asclepiad corresponds to the iambic tetrameter.

As Dale has noticed, [14] we have the attestation of a striking instance showing a poet’s actual perception of the insertion-principle operative {44|45} in the relationship between Glyconic and Asclepiad. Consider fragment 223N of Sophokles: Besides their being morphologically parallel, χρυcόδετον and χορδοτόνου share (1) the same number of syllables, (2) juxtaposition with a following disyllabic word, (3) the consonants χ ρ δ τ. Another sign of poetic artifice seems to be the twice-over occupation of the rhythm-breaking Aeolic base ⏓ ⏓ by a word which actually means ‘break’, ῥηγνύc. I propose that there is at least one other attested instance where a word for ‘break’ seems to have reinforced its own meaning via its position. In verse 9 of the famous Sapphic poem (31LP) that begins φαίνεταί μοι κῆνοc, the expression γλῶccα ἔαγε displays a hiatus otherwise intolerable in this Lesbian genre. The ἔαγε should not be deemed corrupt on that account. [15] Rather, hiatus is the very factor that creates the special effect, namely, that the form is arranged in such a way that it symbolizes what it means. [16]

The internal expansion of Glyconics could {45|46} become reinterpreted synchronically: 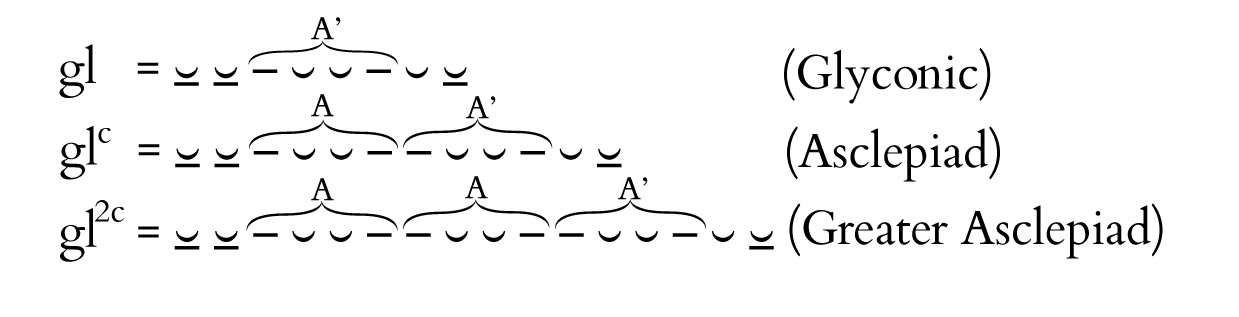We have just derived the sequences labeled by bracket A from the closing of a choriambic dimeter. Notice, however, that the same rhythmical sequence is already present in the Glyconic, as labeled by bracket A’. From a diachronic point of view, we may say that A is a real choriamb, but not A’. From a synchronic point of view, however, A’ = A. In this connection, the following three lyric fragments are crucial:

Scansions 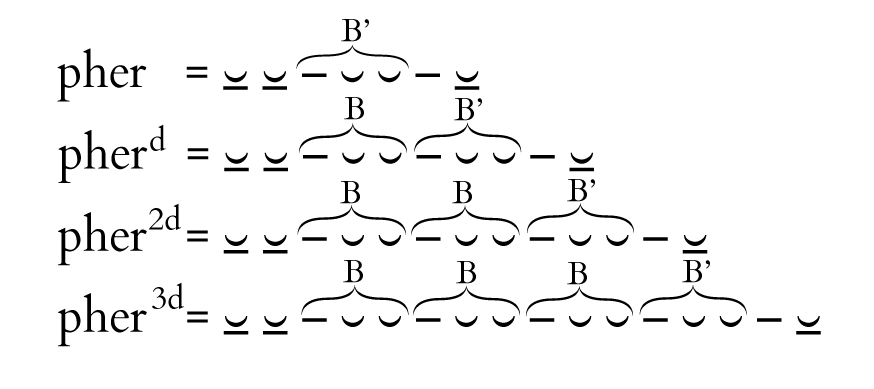These types 1/2/3 are simply Pherecratics with the {46|47} insertion of one/two/three rounds of – ⏑ ⏑ respectively between the Aeolic base (⏓ ⏓) and the fixed segment (– ⏑ ⏑ – ⏓). In line with Snell’s convention, [17] I call these verses pher^d/pher^2d/pher^3d respectively: Just as the Pherecratic equals the Glyconic minus the last syllable, so also the segment – ⏑ ⏑ equals – ⏑ ⏑ – minus the last syllable. The metrical segmentation B’ in Pherecratics is a formal analogue to the symmetrical effect achieved by the metrical segmentation A’ in Glyconics: 2 + 4 + 2 and 2 + 3 + 2, where 4 = A’ and 3 = B’. The A’ has the acoustic shape of a choriamb (– ⏑ ⏑ –), while B’ is what we call a dactyl (– ⏑ ⏑ = d). To sum up: on the model of the internal choriambic expansions of the Glyconic, the Pherecratic could have generated internal dactylic expansions.

My theory that the dactyl originated from the Pherecratic helps account for the fact that there are no attestations of Pherecratics with choriambic expansion. Note, however, that Glyconics with dactylic expansion do exist. The cause, I believe, is that the rhythmical sequence {47|48} of a dactyl is already present in a Glyconic. Once the dactyl arose from the Pherecratic, the Glyconic could be heard as consisting of Aeolic base plus two dactyls. Accordingly, internal expansion may involve not just choriambs but also dactyls : 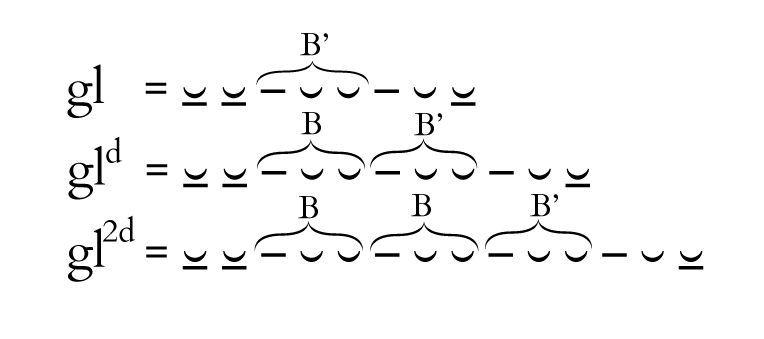(Footnote [18] refers to the second line; footnote [19] to the third.)